Fractals from Nature Into Art

Romanesco Broccoli. This variant form of cauliflower is the ultimate fractal vegetable. Its pattern is a natural representation of the Fibonacci or golden spiral, a logarithmic spiral where every quarter turn is farther from the origin by a factor of phi, the golden ratio. [o]

At the start of his groundbreaking work, The Fractal Geometry of Nature, the mathematician Benoit Mandelbrot asks: "Why is geometry often described as cold and dry? One reason lies in its inability to describe the shape of a cloud, a mountain, a coastline or a tree."

His observation acknowledges the fact that the natural world is not solely made up of the circles, spheres and straight lines — which form the basis of the geometry described by the ancient Greek mathematician Euclid — it also includes rough-edged and irregular shapes.

Taking into account all the jagged edges and tiny nooks and crannies, it was almost impossible to arrive at a precise measurement.

In an attempt to describe the kind of fragmented patterns that are found within nature, Mandelbrot coined the term “fractal” in 1975, basing it on the Latin word fractus, meaning "broken" or "fractured". He defined a fractal as: "a rough or fragmented geometric shape that can be split into parts, each of which is, at least approximately, a reduced-size copy of the whole." Another definition describes a fractal as "a curve or geometric figure, each part of which has the same statistical character as the whole."

Mandelbrot took a keen interest in the appearance of coastlines after reading a book by the British mathematician Lewis Fry Richardson, who had set out to measure the coastline of Britain in the early 1950’s. Taking into account all the jagged edges and tiny nooks and crannies, Richardson concluded that it was almost impossible to arrive at a precise measurement. This conundrum is sometimes known as the coastline paradox.

In 1980, while working as a researcher at IBM, Mandelbrot made use of the company’s powerful computers to generate an enhanced digital image of a complex fractal. This followed the publication of an academic paper by mathematicians Robert Brooks and Peter Matelski two years earlier — sketching out the first rough plot of the same fractal pattern based on the quadratic equation (z n+1 = z n2 + c). Mandelbrot’s version appeared on the cover of the August 1985 issue of Scientific American, later becoming known as the Mandelbrot Set..

This bulbous shape has a complex boundary, which, when viewed in close-up, is composed of endless intricate details: from regular geometric patterns to outlines that have been compared to elephants and seahorses.

A Gallery of Mandelbrot Sets

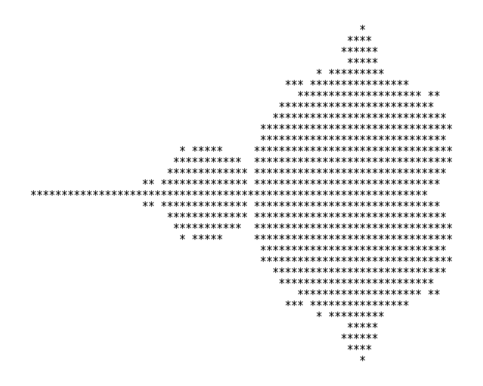
The first published picture of the Mandelbrot set, by Robert W. Brooks and Peter Matelski in 1978. [o]

[o

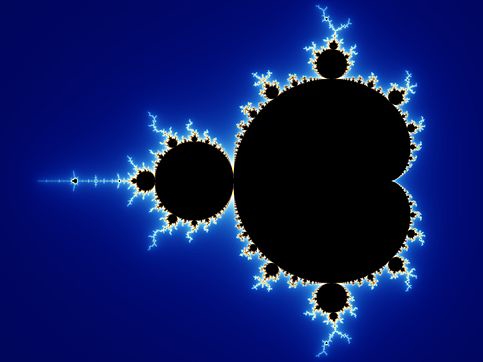
The Mandelbrot set within a continuously coloured environment. [o]

Diving into the Mandelbrot Set — which "exhibit an infinitely complicated boundary that reveals progressively ever-finer recursive detail at increasing magnifications." [o]

Computer scientists soon went on to explore a variety of practical applications for fractal geometry, from the study of marine organisms to modelling flashes of lightning. Computer generated imagery (CGI) uses fractals to create copies of natural landscapes which are almost as good as the real thing, if not quite. This makes the technique ideally suited to the creation of imaginary landscapes required for science fiction and fantasy movies.

From the mid-1980s forward, generating geometric patterns with the aid of algorithms and specialized computer software developed into an art form. The computer artist Kerry Mitchell published a manifesto in 1999 defining fractal art as possessing the following qualities:

1. EXPRESSIVE — Fractal art is just as expressive of an artist’s emotions and thoughts as any other artistic field, despite using algorithms.
2. CREATIVE — Fractal art begins as a blank canvas and develops into a piece of art through the artist’s creative input.
3. INPUT — To create the art, a fractal artist must input formulas, colour schemes, and mappings.
4. ART — Beyond the nuances, fractal art is simply a work created by a fractal artist, not unlike what photographers, painters, and musicians do.

In the same year, American poet Alice Fulton mentioned fractals in a series of essays entitled Feeling as a Foreign Language, highlighting the importance of asymmetry in 20th century aesthetics: “The fascination of these intricate forms indicates that we don’t need an obvious or regular pattern to satisfy our aesthetic or psychological needs. Non-objective art, which often reflects the fractal pattern of nature, makes the same point.”

How we recognize that if we look at fractals our stress is reduced leads to the possibility of creating retinal implants that mimic the phenomenon. [o]

Fractal poetry emerged as an innovative way to blend the written word and images, creating a multi-layered art form. Teacher and poet Marian Christie, who is originally from Harare, Zimbabwe and now lives in Kent, England, writes a blog on mathematical poetry and has published a booklet entitled Fractal Poems. The collection is illustrated with an image of tree branches silhouetted against the sky, generating irregular patterns, as described in her poem Fractal Sunset

   silhouette of oak
   a cloud-patterned sky
   self-similarity
   at every scale

Her poem 'Tree of Pythagoras' is a construction of squares and Isosceles triangles, modelled on a fractal discovered by the Dutch engineer Albert Bosman in 1942. 'Circles within Circles' consists of encircled descriptions of ripples in a pond, icy rings of Saturn, and fairy rings in grass.

The three lines of her poem 'At the Edge' follow the curves of a spiral pattern. Spirals found in nature range in scale from a tiny seashell to a huge galaxy, allowing plenty of scope to develop an endless fascination with fractal forms. ō
 

LINKS

The Fractal Foundation

Lewis Fry Richardson (1881-1953)

Benoit Mandelbrot (1924-2010)

Pioneer fractal artist: Carlos Ginzburg

Other well-known fractal artists, since the 1980s

Software for creating your own fractal art

ANGELA LORD is a freelance writer with a degree in Modern Languages and a background in print journalism and the Nature Editor of The Journal of the Wild Culture. Originally from South Yorkshire in the UK, she is now based in Surrey.